White paper :
The Lost Art of Interest Calculation
|
Abstract Over the last 20 years, computers have eliminated the need to understand basic interest principles. Consequently, most organizations, large and small, have forgotten many fundamentals, and the people who should know what standards and principles apply to their firm’s interest calculations rely on their computers and, very often, older software, which may be incapable of dealing with today's complex market requirements. The article deals with the fundamentals of applied interest calculation that include simple, compound, effective rate, add-on interest calculation methods, compounding period, repayment principles, day count (Actual/actual, 30/360, Actual/365, Actual/360), annual percentage rate (APR) and more complex issues such as the number of weeks in a year, the weight of a month in a year and the non-negligible effect of leap year in high-stakes calculations. |
 |
More than Math
The Lost Art of Interest
Calculation
Nothing seems simpler or less interesting than calculating interest. As well as your trusty calculator, there are dozens of software packages that calculate interest on loans and mortgages. Yet, surprisingly, few of these packages provide truly precise results. People who compare their bank’s numbers with those derived via free or inexpensive software are always surprised to find that they are not the same. Why is this? Isn't there a standard mathematical formula which everyone uses and provides the right numbers? The answer is quite simple: Yes and no.
The heart of the problem is that, over the last 20 years, computers have eliminated the need to understand basic interest principles. Consequently, most organizations, large and small, have forgotten many fundamentals, and the people who should know what standards and principles apply to their firm’s interest calculations rely on their computers and, very often, older software, which may be incapable of dealing with today's complex market requirements.
Laws such as the U.S. Truth in Lending Act , European Directives (Directive 98/7/CE) and other national and regional laws and "customs" provide some guidance, but they leave many blanks. Accordingly, this article is meant to give people who depend on interest for their livelihood (bankers, mortgage brokers other lenders) and those to whom interest is important (real estate professionals, lawyers, accountants and other financial services providers) a comprehensive survey of the intricacies involved and the discrepancies that may occur in the calculation of interest. The text is thus divided it into three sections: Basic basics, Intermediate basics and Advanced basics.
Basic basics
Simple or compound interest?
Most normal loans use compound interest. That is, interest is paid on the interest itself.
In business, simple interest (no interest on interest) is still present for short duration loans and even in longer loans but is not the norm. Simple interest is found in the legal arena, as when interest is payable in liability matters, but the courts are increasingly considering compound interest, which more accurately reflects "real life."
Compounding period
The frequency with which interest is compounded varies according to the type of loan, certain legislation and industry practice. The charged interest rate (or effective rate) is highest when the compounding period is daily and decreases as the period lengthens: weekly, bi-weekly, bimonthly, monthly (mortgages in the US and in most countries), quarterly, semi-annually (mortgages in Canada), annually.
In a loan contract, the "nominal" interest rate is usually the only one quoted. The compounding period then determines the effective (or "real") rate. By definition, the effective and nominal rates will be the same when the compounding period is one year. Quoting effective rates would, of course, give consumers a more accurate idea of a loan's cost. (However, we have not yet discussed, the "truer true" interest rate known as the annual percentage rate (APR), which takes loan fees into account.)
Effective Rate method vs. Capitalized simple interest
Compound interest may be computed in different ways. The most common confusion stems from the effective rate method (some may call it actuarial method but there is controversy at this level since countries will use different terms) and the capitalized simple interest.
Capitalized simple interest is an easy method of computing interest (often used in spreadsheets or with pocket calculators) but is not always fair for the lender and borrower. With this method, the same amount of interest is computed daily throughout the period and at the point of capitalization (compounding) the total interest generated is added to the principal. This balance becomes the new principal upon which interest is computed until the next capitalization.
In contrast, the effective rate method of calculating interest computes interest on a day-by-day basis (as opposed to a lump sum of interest at the capitalization date) using the effective interest rate. Based on an exponential formula, interest earned daily at the start of a period is less than that earned daily later on in the loan.
In loans, with the capitalized simple interest method, the borrower pays more interest in the early months than would have been paid using the effective rate method. The effective rate method treats both the lender and the borrower fairly and an increasing number of jurisdictions have and are requiring that consumer loans use this method.
Interesting note, interest compounding annually with either method will yield, at the loan anniversary date (which is the capitalization date with the capitalized simple interest method), exactly the same total amount of interest.
Principal vs interest
The basic rule in any type of loan, whether compound or simple interest is used, is that accumulated interest is always repaid before the principal. Only when the payment is greater than the total accumulated interest will the principal portion be reduced.
I have seen situations in which lenders wished to find an interest calculation tool that would produce amortization schedules by which interest accumulates and generates interest (i.e., compounds) but payments are first applied against the principal, leaving some interest "hanging." This unusual method although conceivable, is not a recognized norm.
However, the effective rate method does allow repayment methods such as "interest only" (thus no reduction in principal) and "fixed principal" in which a fixed amount of principal is repaid as well as the accumulated interest. In these two methods, the payments are not even and diminish over time.
What about that unusual method called add-on interest?
Also called "pre-calculated" or "pre-computed" interest or the "flat-rate method", the total interest is "added on" to the original principal and the result is simply divided by the number of payments to be made. This makes for simple calculations and is often used in vehicle finance, but the buyer isn't always aware that the interest charged is almost double the stated rate.
For example, a rate of $12.50 per hundred per year on a $20,000 loan to be repaid in monthly payments over 4 years will give us a total add-on of $10,000 (20,000/100 X 12.50 X 4). The total loan is thus $30,000 to be repaid in 48 equal payments at "0.00%" interest. The effective rate becomes 21.5273%.
Rule of 72, Rule of 78
Since these rules were created before the advent of the computer and are now outdated, the article will not deal with these "shortcut" methods. These calculation methods should no longer be used.
Start date, maturity date
In a loan, it may sometimes be difficult to decide whether to include the final (or maturity) day in the interest calculation. Generally, subject to a few exceptions in certain countries, the maturity day is not counted when calculating the days of a loan –- only the elapsed number of days is counted. The logic is quite simple, as illustrated by this example. How much interest is payable on a loan of $10,000 for one day? With a start date of January 1, 2005, say, should the end date be January 1, 2005, or January 2? The latter is more logical, since it takes into account all of January 1 to midnight minus one second, and only one day's interest is payable.
Intermediate Basics
Day count
The day count is the method by which the number of days between two dates (loan date, payment (coupon) dates, end date) is counted. All loans, mortgages and bonds must specify which day count is to be used. It seems simple but there are no less than 20 day count methods, depending on country, industry, client type and type of financial instrument!
The four most common day count methods are outlined below. Depending on country and industry, there may be variants in the names used. These names follow the ISO 15022 nomenclature:
- Actual/actual, sometimes known as actual/365 (ISDA), is the most intuitive and precise day count scheme. To determine the number of days between any two dates, the actual number of days, including the effect of a leap year, are counted. This method is used for treasury bonds and notes, and unless otherwise indicated by law, contract or "custom," it should be used for maximum precision.
- The 30/360 method, also known as "bond basis," was invented in the days before computers to make computations easier. In this method, all months, including February, have 30 days, and all years have 360 days. In the U.S., 30/360 is used for corporate bonds, U.S. agency bonds and mortgage-backed securities.
- The Actual/365 (fixed) method counts the actual number of days of a loan, but the denominator (used to calculate a daily interest rate) will exclude the extra day in a leap year. Thus, a daily interest rate, is always the yearly rate divided by 365.
- Actual/360 is a slightly odd method, which counts the actual number of days during which a loan is outstanding and calculates the interest rate based on a 360-day year. So, over one year, a $1,000 loan at 10% will yield $101.39 in interest (365/360) as opposed to the true $100 return. This type of count obviously benefits the lender.
Annual percentage rate (APR) and Annual Percentage Yield (APY)
The APR is a "standardized" rate (expressed as a percentage), which takes into account all compulsory fees associated with a loan. It is commonly used to compare loan programs offered by different lenders. The Truth in Lending Act requires non-commercial loan and mortgage companies to disclose the APR when they advertise a rate.
The APR expresses the real cost of borrowing. It also considers when fees are paid. Fees paid at the beginning of a loan will have a different effect on the APR than those paid at the end or monthly. Some fees are financed by the loan and will generate additional interest. This also has to be taken into consideration, when calculating the APR. If lump sums are paid, they will also affect the APR, as they lower the interest charges.
There are many interest-calculation software packages out there, lots of interest-calculation methods for amortization and, unfortunately, various APR formulae. The differences in the results obtained via these methods are usually quite small and, one hopes, within the acceptable 1/8% to 1/4% (irregular transaction) accuracy usually required by law.
In some countries, such as France, the law stipulates tough sanctions on inaccurate APR disclosure: the interest on consumer loans being reduced to 0% and the application of a statutory interest rate on commercial loans. Conclusion: don't mess up the APR calculation!
Unfortunately, there are many types of APR: actuarial, US Rule, nominal, effective, real and historical. Thus, it's not always easy to navigate these waters. In the US, there has been some effort to standardize APR, but resistance is strong, because changing something that is already confusing may confuse consumers even more. In Europe, tougher standards have been applied where a true APR must be used: the "effective APR" that takes into all fees as well as the compounding period. The effective APR is in fact known as Annual Percentage Yield or APY, the TRUE interest rate that takes into account fees and compounding.
Advanced Basics
This section concerns subtleties that may produce minor differences in interest totals, often within the allowable error margins. Far too often, the cause of such discrepancies is that the calculation software being used is incapable of handling these subtleties.
Calculating periods in a year
The math behind interest calculation and amortization schedules requires that the number of periods in a year be known. This may seem quite straightforward, but there are a few areas of confusion.
Number of weeks in a year
In calculating amortization when the payment or the compounding period is weekly, biweekly or every four weeks, different software packages will use different numbers of periods per year.
For example, in a 365-day year, weekly payments or compounding may occur 52 times per year or 52.143 times (365/7) or even 52.286 (366/7) times.
Although there are few norms and the differences between the results are quite small, software should include these options.
Relative weight of months
Logic tells us that one month is 1/12 (0.0833) of a year and the interest for a full month should be this fraction.
Another possibility is to base the interest on the number of days in each month. By this method, January would count for slightly more than one-twelfth: 31/365 (0.0849) -- or 31/366 (0.0847) in a leap year, if the day count is actual/actual). So, what about February? Should less interest be paid in February than in a 31-day month? Using this method, yes.
Generally, in regular calculations with regular payments, the first method is more widely used, but both methods are acceptable.
Denominator year basis
When using the actual/actual method of simple interest calculation to determine the interest on a loan or a period of a loan (formula: principal balance X yearly interest rate X number of days/number of days in a year), leap year has a subtle effect on the "number of days" (the denominator). Various methods are used to determine whether it will be 365 or 366 days.
One method divides the calculation to take into account, as a reference, the civil year. The example in Table One illustrates the calculation method by which the interest accumulated from Nov. 1, 2003, to Dec. 1, 2003, is principal (or balance) X yearly interest rate X 30/365. In the bond market, this is called the ISDA method (International Swaps and Derivatives Association).

Table One: Civil year method (ISDA)
A second method, slightly more complicated, takes into account the payment anniversary date to calculate the base year. The example in Table Two shows the calculation method by which, counting backwards, the number of days are taken from the end of the actual payment period to a year prior. For the interest accumulated from Nov. 1, 2003, to Dec. 1, 2003, this method will take into account the anniversary date (December 2003 back to December 2002), to calculate the number of days in that year. Since there are 365 days in this period, 365 will be used as the base. For the March 1, 2004, to April 1, 2004, period, the 366-day basis will also be used, because from April 1, 2004 to April 1, 2003 (counting backwards), there are 366 days.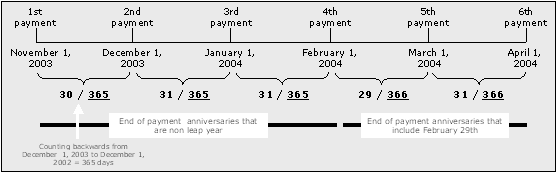
Table Two: Anniversary method
In the bond market, other methods include ISMA (International Securities Markets Association) and the AFB (Association Française de Banques), which borrow aspects of the above methods but with certain subtleties that will not be looked at here.
Irregular payments become simple interest
Finally, an oddity, which probably reflects a lack of proper calculation tools in the distant past. In some jurisdictions, when a payment on a regular compound interest (effective rate) loan is late or otherwise irregular, the simple interest method is used to calculate the interest for the arrears period.
For example, if a payment due on the 1st of a month is not paid until the 12th, interest would be calculated this way: balance X daily rate X 12/31) + (balance X daily rate X 19/31). The effective rate method, on the other hand, would continue to calculate the interest normally for this period. Although historically understandable, and the difference between both methods is quite small, I find this sudden change of method clumsy and difficult to justify in the information age.
Conclusion
This survey has highlighted the sources of the widespread confusion I have observed over many years of studying applied interest principles, working with clients and seeing what the market has to offer in terms of solutions. It is hoped that, when the numbers just don't add up, these basics will steer the professional dealing with interest toward accurate and satisfying solutions.
When legislation does not specifically provide a solution, one should be aware of the possibilities and where discrepancies may lie. Moreover, to ensure maximum precision in interest calculations, the elements outlined above should be included in the calculation parameters, as is done in major international transactions.
Bibliography:
About the author
Marc Gelinas, LLB, MBA, is the founder and CEO of Jurismedia, Inc., which publishes Margill, an interest-calculation software tool used by thousands of accountants, bankers, mortgage brokers, lease professionals, attorneys, judges, trade unions and financial planners. As a lawyer, he has, over the last 15 years, been called upon to resolve many complex issues dealing with interest and its accuracy in the U.S., Canada and Europe.
Jurismedia inc.
Tel.: 1-877-683-1815
Direct: (450) 621-8283
Fax: (450) 621-4452
Email: [email protected]
US office:
220 E. Delaware Avenue
Newark, Delaware
19711
U.S.A.
75, boul. des Châteaux,
Lorraine, Quebec
Blainville, Quebec J7B 2A4
Canada
Copyright ©, 2006-2009, Marc Gelinas
To reproduce or use any part of this White Paper, please contact the author.
Last revision: February 2009